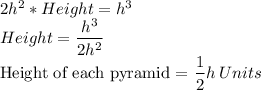Answer:
(A)The height of each pyramid is One-half h units.
Explanation:
Height of the Cube = h units
Volume of the Cube
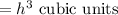
If Base of the cube =Base of the square pyramid
Base of the square pyramid = h units
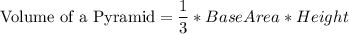
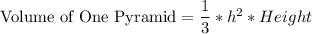
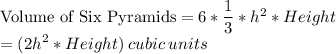
Since Volume of the Cube = Volume of Six Square Pyramids
Then: