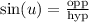1 Answer

Usealbarazer · Answer 1 · 2021-01-15T07:17:33+0000

Answer:

(x)/(√(x^2+9))

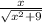
Explanation:

Let
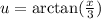$u=\arctan((x)/(3))$ .

Then
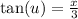$\tan(u)=(x)/(3)$ .

Construct a right triangle.

Make one of the non-right angles
.

Recall
$\tan(u)$ is equal to the ratio of the side that is opposite to
to the side that is adjacent to
.

This means opposite side
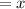while adjacent side
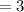since
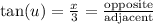$\tan(u)=(x)/(3)=\frac{\text{opposite}}{\text{adjacent}}$ .

We can find the hypotenuse by using the Pythagorean Theorem.

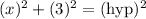
$(x)^2+(3)^2=(\text{hyp})^2$

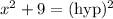
$x^2+9=(\text{hyp})^2$

Take the square root of both sides:

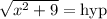$√(x^2+9)=\text{hyp}$ .

Recall
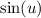$\sin(u)$ is equal to the ratio of the side that is opposite to
to the hypotenuse.

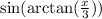
$\sin(\arctan((x)/(3)))$

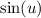$\sin(u)$ -->Because we let the inside equal
.

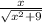(x)/(√(x^2+9)) -->Because
$\sin(u)=\frac{\text{opp}}{\text{hyp}}$

Please log in or register to add a comment.