Answer:
the height of packing required for this separation is 15.85 m
Step-by-step explanation:
Given that :
liquid stream (A+C) enters a packed column which the solute is stripped by using a pure gas B
The solute flowrate
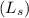 = 150 kmole/hr
= 150 kmole/hr
Mole fraction of the solute
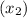 = 7% = 0.07
= 7% = 0.07
(A+C)liquid =
 = 0.01
= 0.01
Pure gas
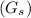 = 500 kmole/hr
= 500 kmole/hr

The delivery force in the gas phase & liquid phase is expressed as:
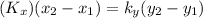
Given that
 = 75 kmole/hr m³ and equilibrium relationship
= 75 kmole/hr m³ and equilibrium relationship
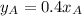
Then :

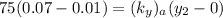
where ;
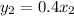
= 0.4(0.07)
= 0.028
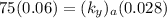

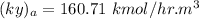
NOW; the overall mass transfer coefficient on the liquid phase is :-
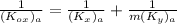
where m= slope = 0.4

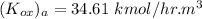
Finally; the height of the tower (z) is =

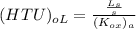
where :
s = 1m²
 = 150 kmol/hr
= 150 kmol/hr
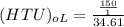 = 4.33 m
= 4.33 m
![(NTU)_(oL) = e^x [(((x_2-(y_1)/(m) )/(x_1-(y_1)/(m) ))(1-A)+A )/(1-A) ]](https://img.qammunity.org/2021/formulas/engineering/college/4e9u8x82m8rq5rp8tdbdizpm5natt559tx.png)
where A =
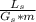 =
=
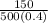
= 0.75
Then:
![(NTU)_(oL) = e^x [(((0.07-0 )/(0.01-0 ))(1-0.75)+0.75 )/(1-0.75) ]](https://img.qammunity.org/2021/formulas/engineering/college/gllr85v3w3viry1qew9tk9qm77h1hbptm3.png)
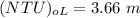
the height of the tower (z) is =

= (4.33)(3.66)
=15.85 m
Thus, the height of packing required for this separation is 15.85 m