Question

A linear function has an x-intercept of 12 and a slope of StartFraction 3 Over 8 EndFraction. How does this function compare to the linear function that is represented by the table?

x
y
Negative two-thirds
Negative three-fourths
Negative one-sixth
Negative StartFraction 9 Over 16 EndFraction
One-third
Negative StartFraction 3 Over 8 EndFraction
StartFraction 5 Over 6 EndFraction
Negative StartFraction 3 Over 16 EndFraction


A It has the same slope and the same y-intercept.
B It has the same slope and a different y-intercept.
C It has the same y-intercept and a different slope.
D It has a different slope and a different y-intercept.

Answer 1

B!

Explanation:

Answer 2

(B) It has the same slope and a different y-intercept

Explanation:

The table is presented below:

`\left|\begin{array}cx&y\\----&---\\-(2)/(3) &-(3)/(4)\\\\-(1)/(6)&-(9)/(16)\\\\(1)/(3)&-(3)/(8)\\\\(5)/(6)&-(3)/(16)\end{array}\right|`

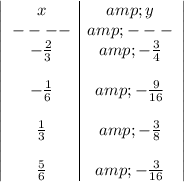
Gradient:

`m=(-(9)/(16)-(-(3)/(4)))/(-(1)/(6)-(-(2)/(3)))\\=(-(9)/(16)+(3)/(4))/(-(1)/(6)+(2)/(3))\\=(3)/(16)/ (1)/(2)\\m=(3)/(8)`

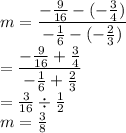
Next, we determine its y-intercept

Using the pair
`(-(2)/(3),-(3)/(4))}` in y=mx+c

`-(3)/(4)=((3)/(8))(-(2)/(3))+c\\-(3)/(4)+(1)/(4)=c\\c=-(1)/(2)`

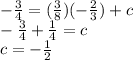
Comparing with the linear function has an x-intercept of 12 and a slope of
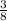`(3)/(8)`, we find out that It has the same slope and a different y-intercept.

Option B is the correct option.