Answer:
a. EPQ = 689.4
b. Np = 17.99
c. Qmax = $575.65
d. %t = 16.54%
e. TC = $1,151.28
Step-by-step explanation:
Let's begin by listing out the variables:
Production per day (P) = 300, Component rate usage (D) for 250 days = 12,400, Holding cost (H) = $2 per item per year, Ordering cost (S) = $32 per order
To calculate the component rate usage per day, we have:
d = D ÷ 250 = 12400 ÷ 250 = 49.6
d/P = 49.6/300 = 0.165
a) EOQ = square root of [(2 times the annual demand in units times the incremental cost to process an order) divided by (the incremental annual cost to carry one unit in inventory)]
EPQ =
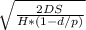
EPQ =
 [2 * 12400 * 32 ÷ 2 (1 - 0.165)]
[2 * 12400 * 32 ÷ 2 (1 - 0.165)]
EPQ =
 [793600 ÷ 1.67]
[793600 ÷ 1.67]
EPQ = sqrt[475209.58] = 689.35
EPQ = 689.4
b) To calculate the production runs per year, we have:
Np = D ÷ EPQ = 12400 ÷ 689.4 = 17.9867
Np = 17.99
c) To calculate maximum inventory level, we have:
Qmax = EPQ * (1 - d/P) = 689.4 * (1 - 0.165)
Qmax = 689.4 * 0.835 = 575.649
Qmax = $575.65
d) Percentage of time the company produces is calculated thus:
%t = (EPQ *Np * 100%) ÷ (P * t)
%t = (689.4 * 17.99 * 100%) ÷ (300 * 250)
%t = 16.54%
e) The annual cost of ordering and holding inventory is calculated thus:
TC = (Np * S) + [(Qmax ÷ 2) * H]
TC = (17.99 * 32) + [(575.6 ÷ 2) * 2]
TC = 575.68 + 575.6
TC = $1,151.28