Question

Suppose that an alien lands on Earth and wants to estimate the proportion of humans who are male. Fortunately, the alien had a good statistics course on its home planet, so it knows to take a sample of human beings and produce a confidence interval. Suppose that the alien happened upon the members of Dr. Cortes’s statistics class from fall semester, so it finds 7 males in its sample (there were a total of 38 students).

Use this sample information (with technology) to form, a 95% one-proportion z-confidence interval for the actual proportion of all humans who are female.

Answer 1

The 95% confidence interval for the population proportion of females is (0.693, 0.939)

Explanation:

We have to calculate a 95% confidence interval for the proportion.

If the sample collected, of size n=38, has 7 males and 31 females, the sample proportion is p=0.8158.

`p=X/n=31/38=0.8158`

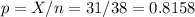
The estimated standard error of the proportion is:

`\sigma_p=\sqrt{(p(1-p))/(n)}=\sqrt{(0.8158*0.1842)/(38)}\\\\\\ \sigma_p=√(0.004)=0.063`

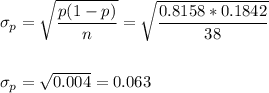
The critical z-value for a 95% confidence interval is z=1.96.

The margin of error (MOE) can be calculated as:

`MOE=z\cdot \sigma_p=1.96 \cdot 0.063=0.123`

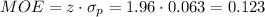
Then, the lower and upper bounds of the confidence interval are:

`LL=p-z \cdot \sigma_p = 0.8158-0.123=0.693\\\\UL=p+z \cdot \sigma_p = 0.8158+0.123=0.939`

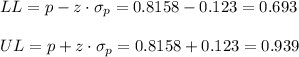
The 95% confidence interval for the population proportion of females is (0.693, 0.939), estimated from this sample.