Answer:
(a)
if
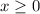 then
then
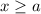 and if x<0 then
and if x<0 then

(b)
That is straightforward from what you showed on theorem 3.25
(c)
Following the same ideas from (a) x>a or -x > a.
Explanation:
Remember how we define the absolute value of a number.
(a)
In general
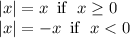
Therefore if
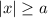 you have two cases, if
you have two cases, if
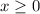 then
then
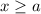 and if x<0 then
and if x<0 then

(b)
That is straightforward from what you showed on theorem 3.25
(c)
Following the same ideas from (a) x>a or -x > a.