Answer:
The vertex of g(x) is (-3, -2).
Explanation:
To find what g(x) truly represents, we must substitute r(x) with the function itself to get the derived function g(x).
g(x) = r(x) - 2
g(x) = x² + 6x + 9 - 2 (Simplify)
g(x) = x² + 6x + 7 (Now, we can start to find the vertex of this quadratic function)
The vertex can be found, when the function is in standard form (AX² + BX + C), by this defined ordered pair:
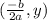
Notice that we already know what A, B, and C are, so we don't need to worry about them, but we don't have the y value. This can be calculated by plugging in the x value for the axis of symmetry which is (-b) / (2a). The vertex is always at the axis of symmetry and so, by plugging in the x value for the axis of symmetry, we can find the exact y value for the location of the vertex on the cartesian plane.
A = 1, B = 6, C = 7 in the quadratic function g(x).
The x value for the vertex can be found by:
x = (-6) / 2(1) (Simplify)
x = -3
Now that we have x, we can find y by substituting this into g(x):
g(x) = (-3)² + 6(-3) + 7 (Simplify)
g(x) = 9 - 18 + 7 (Simplify)
g(x) = -2
The y value for our vertex is -2.
The vertex of g(x) is (-3, -2).