Question

A teacher was interested in finding out whether a special study program would increase the scores of students on a national exam. Fourteen students were selected and paired according to IQ and scholastic performance. One student from each pair was randomly selected to participate in the special program, with the other student participating in the standard program. Both programs ended at the same time. Shortly thereafter, the students took the national exam. The results were as follows: Special program Standard program Descriptive statistics for two-sample test Z Variable Special Program Standard Program Mean 77.571 75.429 StDev 10.454 9.947 7 Descriptive statistics for paired test Variable Special - Standard N Mean 7 2.14 StDev 2.79 At a 5% significance level, is there sufficient evidence to indicate that the special study program is more effective in raising the national exam scores, on the average? Assume approximate normality for the population of differences.

Answer 1

Check the explanation

Explanation:

Going by the first attached image below we reject H_o against H_1 if obs.
`T > t_(\alpha /2;n-1)`

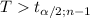
here obs.T=1.879

`\therefore obs.T \\gtr 2.447=t_(0.025;6)`

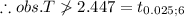
we accept
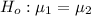`H_o:\mu _(1)=\mu_(2)` at 5% level of significance.

i.e there is no sufficient evidence to indicate that the special study program is more effective at 5% level of significance.

1.

this problem is simillar to the previous one except the alternative hypothesis.

Let X_i's denote the bonuses given by female managers and Y_i's denote the bonuses given by male managers.

we assume that
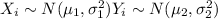`X_i \sim N(\mu _(1),\sigma _(1)^(2)) Y_i \sim N(\mu _(2),\sigma _(2)^(2))` independently

We want to test
`H_0:\mu_(1)=\mu_(2) vs H_1:\mu_(1)\\eq \mu_(2)`

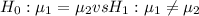
define
`D_i=X_i-Y_i , i=1(1)8`

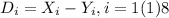
now
`D_i\sim N(\mu _(1)-\mu _(2)=\mu _(D),\sigma _(1)^(2)+\sigma _(2)^(2)=\sigma _(D)^(2)) , i=1(1)8`

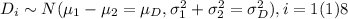
the hypothesis becomes

`H_0:\mu_(D)=0 vs H_1:\mu_(D)\\eq 0`

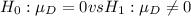
in the third attached image, we use the same test statistic as before

i.e at 5% level of significance there is not enough evidence to indicate a difference in average bonuses .