Question

A cellphone provider has the business objective of wanting to estimate the proportion of subscribers who would upgrade to a new cellphone with improved features if it were made available at a substantially reduced cost. Data are collected from a random sample of 500 subscribers. The results indicate that 135 of the subscribers would upgrade to a new cellphone at a reduced cost.

a. At the 0.05 level of significance, is there evidence that more than 20% of the customers would upgrade to a new cellphone at a reduced cost?
b. How would the manager in charge of promotional programs concerning residential customers use the results in (a)?

Answer 1

a) There is evidence that more than 20% of the customers would upgrade to a new cellphone at a reduced cost

b) The manager in charge of promotional programs concerning residential customers will help the customers to upgrade their cellphones at a reduced cost.

Explanation:

sample size, n = 500

The number of subscribers who would upgrade to a new cellphone at reduced cost, X = 135

Proportion of those that would upgrade to a new cellphone,

`\hat{p} = (X)/(n) \\\hat{p} = 135/500\\\hat{p} = 0.27`

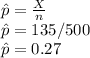
The null hypothesis is that less than or equal to 20%( i.e. 0.2) of the customers would upgrade to a new cellphone at a reduced cost while the alternative hypothesis is that more than 20% would upgrade to a new cellphone at a reduced cost.

Null hypothesis,
`H_(0) : p \leq 0.20`

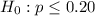
Alternative hypothesis,
`H_(a) : p > 0.20`

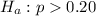
The test statistic can be calculated as thus:

`z_(test) = \frac{\hat{p} - p_(0) }{\sqrt{(p_(0)(1 - p_(0)) )/(n) } }`

`z_(test) = \frac{0.27 - 0.20}{\sqrt{(0.20(1 -0.20))/(500) } }`

`z_(test) = 3.913`

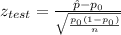
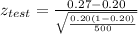
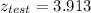
To get the p - value for the test statistic:

`p(z > 3.913) = 1 - p(z \leq 3.913)\\p(z \leq 3.913) = 0.999954 ( from the distribution table)\\p(z > 3.913) = 1 - 0.999954\\p(z > 3.913) =0.000046`

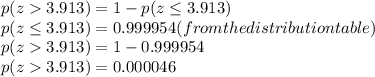
Since the p - value is less than 0.05 significance level, the null hypothesis will be rejected. (i.e. more than 20% would upgrade to a new cellphone at a reduced cost.)