Rewrite the boundary lines y = -1 - x and y = x - 1 as functions of y :
y = -1 - x ==> x = -1 - y
y = x - 1 ==> x = 1 + y
So if we let x range between these two lines, we need to let y vary between the point where these lines intersect, and the line y = 1.
This means the area is given by the integral,
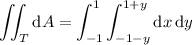
The integral with respect to x is trivial:
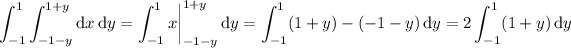
For the remaining integral, integrate term-by-term to get
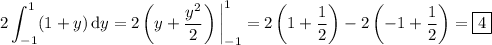
Alternatively, the triangle can be said to have a base of length 4 (the distance from (-2, 1) to (2, 1)) and a height of length 2 (the distance from the line y = 1 and (0, -1)), so its area is 1/2*4*2 = 4.