Relative to the circle with the equation x +y = 4, how has the circle with the equation (x + 5)2+(-6) = 4 been shifted? What is the radius of the circle?

Question

asked Oct 13, 2021 207k views

1 Answer

Vishnu Mishra · Answer 1 · 2021-10-18T14:12:20+0000

Answer:

Circle (ii) shifted 5 units left and 6 units up to get the circle (iii).

The radius of the circle is 2 units.

Explanation:

The standard form of a circle is

(x-h)^2+(y-k)^2=r^2 ...(i)

where, (h,k) is center and r is radius of the circle.

Consider the given equations of the circle are

x+y=4 ...(ii)

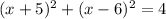(x+5)^2+(x-6)^2=4 ...(iii)

From (i) and (ii), we get

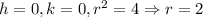
$h=0,k=0,r^2=4\Rightarrow r=2$

So, the center of circle (ii) is (0,0) and radius is 2.

From (i) and (iii), we get

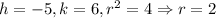
$h=-5,k=6,r^2=4\Rightarrow r=2$

So, the center of circle (iii) is (-5,6) and radius is 2.

Therefore, the circle (ii) shifted 5 units left and 6 units up to get the circle (iii). The radius of the circle is 2 units.