Answer:
The probability that a randomly chosen light bulb will last less than 900 hours is 0.1587.
Explanation:
The life span of these light bulbs is normally distributed with a mean of 1000 hours and a standard deviation of 100 hours
Mean =

Standard deviation =

We are supposed to find the probability that a randomly chosen light bulb will last less than 900 hours.i.e. P(x<900)
So,

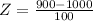
Z=-1
P(x<900)=P(z<-1)=0.1587
Hence the probability that a randomly chosen light bulb will last less than 900 hours is 0.1587.