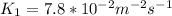Question

A mixture of XO2 (P = 3.00 atm) and O2 (P = 1.00 atm) is placed in a container. This elementary reaction takes place at 27 °C: 2 XO2 (g) + O2 (g) k1 k−1 2 XO3 (g) At equilibrium, the total pressure is 3.75 atm. (a) Determine the value of standard free energy ∆G◦ rxn at 27 °C (unit: kJ/mol). (b) Given that k1 = 7.8 × 10−2 M−2 s −1 , determine the value of k−1 at 27 °C (unit: M−1 s −1 ).

Answer 1

a)
`\triangle G^(0) = 7.31 kJ/mol`

b)
`K_(-1) = 0.0594 m^(-1) s^(-1)`

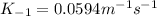
Step-by-step explanation:

Equation of reaction:

`2 XO_(2) (g) + O_(2) (g) \rightleftharpoons 2XO_(3) (g)`

Initial pressure 3 1 0

Pressure change 2P 1P 2P

Total pressure = (3-2P) + (1-P) + (2P)

Total Pressure = 3.75 atm

(3-2P) + (1-P) + (2P) = 3.75

4 - P = 3.75

P = 4 - 3.75

P = 0.25 atm

Let us calculate the pressure of each of the components of the reaction:

Pressure of XO2 = 3 - 2P = 3 - 2(0.25)

Pressure of XO2 =2.5 atm

Pressure of O2 = 1 - P = 1 -0.25

Pressure of O2 = 0.75 atm

Pressure of XO3 = 2P = 2 * 0.25

Pressure of XO3 = 0.5 atm

From the reaction, equilibrium constant can be calculated using the formula:

`K_(p) = ([PXO_(3)] ^(2) )/([PXO_(2)] ^(2)[PO_(2)] )`

`K_(p) = (0.5^2)/(2.5^2 *0.75) \\K_(p) = 0.0533 = K_(eq)`

Standard free energy:

`\triangle G^(0) = - RT ln k_(eq) \\\triangle G^(0) = -(0.008314*300* ln0.0533)\\\triangle G^(0) = 7.31 kJ/mol`

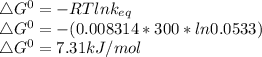
b) value of k−1 at 27 °C, i.e. 300K

`K_(1) = 7.8 * 10^(-2) m^(-2) s^(-1)`

`K_(c) = K_(p)RT\\K_(c) = 0.0533* 0.0821 * 300\\K_(c) = 1.313 m^(-1)`

`K_(-1) = (K_(1) )/(K_(c) ) \\K_(-1) = (7.8 * 10^(-2) )/(1.313 )\\K_(-1) = 0.0594 m^(-1) s^(-1)`