Question

Rimshot was comparing two spheres one with a 30-cm radius and one with a 60-cm radius how many times greater is the volume of the larger sphere

Answer 1

Explanation:

Radius of first sphere is 30 cm.

Volume of first sphere is :

`V_1=(4)/(3)\pi r_1^3\\\\V_1=(4)/(3)* 3.14* (30)^3\\\\V_1=113040\ cm^3`

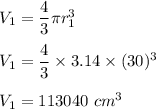
Radius of second sphere is 60 cm.

Volume of second sphere is :

`V_2=(4)/(3)\pi r_2^3\\\\V_2=(4)/(3)* 3.14* (60)^3\\\\V_2=904320\ cm^3`

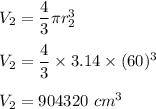
Dividing volume of second sphere to that of volume of first sphere. So

`(V_2)/(V_1)=(904320)/(113040)\\\\(V_2)/(V_1)=8\\\\V_2=8V_1`

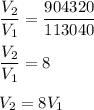
So, the volume of the larger sphere is 8 times of the volume of smaller sphere.