Question

PLEASE HELP ME I WILL LITERALLY GIVE YOU A KISS ON THE CHEEK

Use the function f(x) to answer the questions:

f(x) = 5x2 + 2x − 3

Part A: What are the x-intercepts of the graph of f(x)? Show your work. (2 points)

Part B: Is the vertex of the graph of f(x) going to be a maximum or a minimum? What are the coordinates of the vertex? Justify your answers and show your work. (3 points)

Part C: What are the steps you would use to graph f(x)? Justify that you can use the answers obtained in Part A and Part B to draw the graph. (5 points)

Answer 1

A) x-intercepts are (0.6, 0) and (-1, 0)

B) vertex is (-0.2, -3.2)

C) see attached

Explanation:

Part A

Given function:
`f(x)=5x^2+2x-3`

The x-intercepts are when f(x) = 0

`\implies 5x^2+2x-3=0`

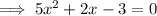
To factor, find two numbers that multiply to -15 and sum to 2: 5 and -3

Rewrite the middle term of the quadratic as the sum of these number:

`\implies 5x^2+5x-3x-3=0`

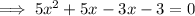
Factorize the first two terms and the last two terms separately:

`\implies 5x(x+1)-3(x+1)=0`

Factor out the common term
`(x+1)`:

`\implies (5x-3)(x+1)=0`

`\implies (5x-3)=0\implies x=\frac35=0.6`

`\implies (x+1)=0 \implies x=-1`

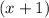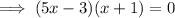
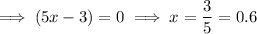
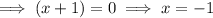
Therefore, the x-intercepts are (0.6, 0) and (-1, 0)

Part B

As the leading coefficient of the quadratic is positive, the parabola will open upwards. This means that the vertex will be a minimum point.

The x-coordinate of the vertex is the midpoint of the zeros.

`\sf midpoint=(-1+0.6)/(2)=-0.2`

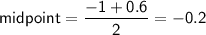
To find the y-coordinate of the vertex, substitute the found value of x into the given equation:

`f(-0.2)=5(-0.2)^2+2(-0.2)-3=-3.2`

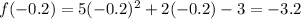
Therefore, the vertex is (-0.2, -3.2)

Part C

Plot the zeros and the vertex.

The axis of symmetry is the x value of the vertex, so ensure that the graph is symmetrical about x = -0.2.