Question

Write an equation of the line that passes through a pair of points:

a. y = x + 3
b. y = x-3
C. y = -x + 2
d. y=-x-2

Answer 1

B

Explanation:

Our equation should be in the form y = mx + b, where m is the slope and b is the y-intercept.

The slope is found by taking any two points on the line and finding the change/difference in the y-coordinates and then dividing that by the change/difference in the x-coordinates.

Here, we're given two points: (4, 1) and (5, 2). Our slope will then be:
`(2-1)/(5-4) =(1)/(1) =1`

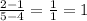
The slope is 1, so m = 1.

The y-intercept is the place where the graph intersects with the y-axis. Looking on the graph, that point is (0, -3), so the y-intercept is b - 3.

Plug these values into the equation:

y = mx + b

y = 1 * x + (-3)

y = x - 3

The answer is B.

Answer 2

Answer choice B

Explanation:

This line passes through the points (4,1) and (5,2). The formula for a line in slope intercept form is y=mx+b, where m represents the slope and b represents the y coordinate of the y intercept. The slope can be found by comparing the rise with the run. When the line goes from (4,1) to (5,2), it rises 1 unit and runs (goes to the right) 1 unit. 1/1=1, so the slope of this line is 1. The y intercept can be seen to be at (0,-3), meaning that b=-3. Therefore, the formula is y=x-3 or answer choice B. Hope this helps!