Answer:
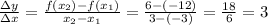
Explanation:
To find the average rate of change of a function over a given interval, basically you need to find the slope. The mathematical definition of the slope is very similar to the one we use every day. In mathematics, the slope is the relationship between the vertical and horizontal changes between two points on a surface or a line. In this sense, the slope can be found using the following expression:
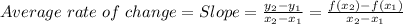
So, the average rate of change of:
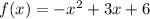
Over the interval
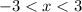
Is:
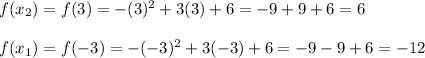
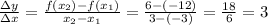
Therefore, the average rate of change of this function over that interval is 3.