Question

. Shanice is making a garden. The area of the garden is 24 ft2. Use the following shape and information to find the base (6−4) and height (+3) of the garden. The equation for area of a triangle is =1/2ℎ.

Answer 1

The base and height of the garden are 4.60 ft and 5.60 ft.

Explanation:

The area of the garden is, A = 24 ft².

The base height of the garden are:

base (b) = 6x - 4

height (h) = x + 3

Compute the value of x as follows:

`A=(1)/(2)* b * h\\\\24=(1)/(2)* (6x-4) * (x+3)\\\\48=6x^(2)+18x-4x-12\\\\6x^(2)+14x-60=0\\\\3x^(2)+7x-30=0\\`

The last equation is a quadratic equation.

Compute the roots of the quadratic equation as follows:

`x = ( -b \pm √(b^2 - 4ac))/( 2a )\\x = ( -14 \pm √(14^2 - 4(3)(-30)))/( 2(3) )\\x = ( -14 \pm √(556))/( 6 )\\x = ( -14 )/( 6 ) \pm (2√(139)\, )/( 6 )\\x = -( 7)/( 3 ) \pm ( √(139)\, )/( 3 )\\x = 1.59661\\x\approx 1.60`

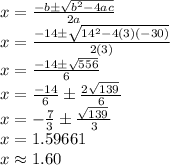
The value of base and height are:

`\text{base}=6x-4=(6* 1.60)-4=5.60\ \text{ft}\\\\\text{height}=x+3=1.60+3=4.60\ \text{ft}`

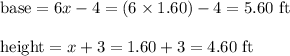
Thus, the base and height of the garden are 4.60 ft and 5.60 ft.