Answer:
Question 1
The function
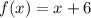 is one-to-one, so it does have an inverse.
is one-to-one, so it does have an inverse.
The inverse of +6 is -6, so
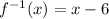
Therefore, g(x) is the inverse of f(x).
Question 2
The function
 is one-to-one, so it does have an inverse.
is one-to-one, so it does have an inverse.
To find the inverse, replace f(x) with y:

Rearrange the equation to make x the subject:
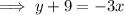
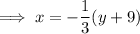
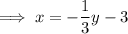
Replace x with
 and y with x:
and y with x:
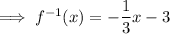
Therefore, g(x) is the inverse of f(x).