Answer:
1.) 20.2
Explanation:
1.) You need to use the distance formula:

Find the distance of A to B first:

B to C:
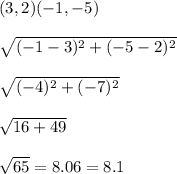
C to A:
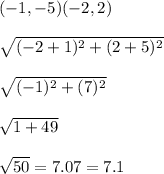
Add distances to find the perimeter:

2.) Part A:
You need to use the mid-point formula:


Part B:
1. Use the slope-intercept formula:

M as the slope, b the y-intercept.
Find the slope of the two points A and B using the slope formula:
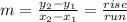
Insert slope as m into equation.
Take point A as coordinates
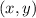 and insert into the equation. Solve for the intercept, b:
and insert into the equation. Solve for the intercept, b:
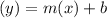
Insert the value of b into the equation.
2. Use the mid-point coordinate. Take the slope.
If you need to find the perpendicular bisector, you will take the negative reciprocal of the slope. Switch the sign and flip it. Ex:
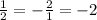
Insert the new slope into the slope-intercept equation as m.
Take the mid-point coordinate as (x,y) and insert into the equation with the new points. Solve for b.
Insert the value of b.