Question

In centimeters, what is the unknown length in this right triangle?

A right triangle with side length 60 centimeters, x, and hypotenuse of 61 centimeters.

Answer 1

• Given:-

Side length ( perpendicular = 60 cm)

Hypotenuse = 61 cm

• To find :-

Base length

• Solution:

By Pythagoras theorem ,

Hypotenuse² = Perpendicular² + Base²

61² = 60² + base²

3721-3600 = Base²

√121 = base

11cm = base

Answer 2

• Base = x = 11 cm

Explanation:

In the question we are given ,

• Side length ( Perpendicular ) = 60 cm

• Hypotenuse = 61 cm

And we have asked to find the length of base that is denoted by x .

Solution : -

According to Pythagoras Theorem ,

`\longrightarrow \purple{\boxed{H {}^(2) = P {}^(2) + B {}^(2) }} \longleftarrow`

Where ,

• H = Hypotenuse

• P = Perpendicular

• B = Base

Now , substituting value of hypotenuse, perpendicular and base :

`\hookrightarrow \: 61 {}^(2) = 60 {}^(2) + x {}^(2)`

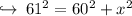
Now by squaring 61 and 60 , we get :

`\hookrightarrow \:3721 = 3600 + x {}^(2)`

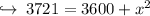
Now transposing 3600 to left hand side :

`\hookrightarrow \:3721 - 3600 = x {}^(2)`

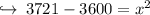
Now subtracting 3600 from 3721 :

`\hookrightarrow \:121 = x {}^(2)`

`\hookrightarrow \: √(121) = x`

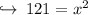
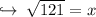
We know that 11 × 11 is equal to 121 that means square root of 121 is 11 . So :

`\hookrightarrow \: \red{ \boxed{11 \: cm = x}}`

• x = base

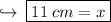
• Henceforth, length of base of right triangle is 11 cm .

#Keep Learning