Question

The value of new cars at a local dealership is normally distributed with a mean of $34,600 and a standard deviation of $3,000. What percentage of new cars would you expect to have a sticker price of between $31,600 and $40,600? (Include the percent sign in your answer without a space.)

Answer 1

The percentage that a new car is expected to have a sticker price of between $31,600 and $40,600 is 81.86%.

Explanation:

The random variable X is defined as the value of new cars at a local dealership.

The mean of the random variable X is, μ = $34,600 and the standard deviation is, σ = $3,000.

The random variable X is normally distributed.

Compute the probability that a new car is expected to have a sticker price of between $31,600 and $40,600 as follows:

`P(31600<X<40600)=P((31600-34600)/(3000)<(X-u)/(\sigma)<(40600-34600)/(3000))\\\\=P(-1<Z<2)\\\\=P(Z<2)-P(Z<-2)\\\\=0.97725-0.15866\\\\=0.81859\\\\\approx 0.8186`

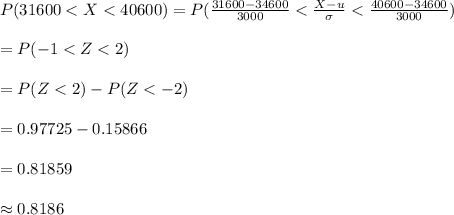
The percentage is, 0.8186 × 100 = 81.86%.

Thus, the percentage that a new car is expected to have a sticker price of between $31,600 and $40,600 is 81.86%.