2 Answers

Jose Elera · Answer 1 · 2021-04-08T14:29:17+0000

Answer:

$5\sqrt[]{6}$

Explanation:

$\sqrt[]{54}+\sqrt[]{24}$

We can rewrite 54 as 6*9 and 9 can be rewritten as
3^2 .

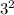24 can be rewritten as 3*8 and 8 can be rewritten as
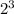2^3 or
2^2*2

I'm doing this because that way I can easily take those numbers out of the square (2) root.

Let's rewrite our square roots.

$\sqrt[]{6*3^2}+\sqrt[]{3*2^2*2}$

3 cancels the square in the first square root, and same does 2 squared in the second square root.

$3\sqrt[]{6}+2\sqrt[]{3*2}$

Solve;

$3\sqrt[]{6}+2\sqrt[]{6}$

When you have square roots with equal value inside, you can add the numbers outside of it.

$3+2(\sqrt[]{6})$

$5\sqrt[]{6}$

Please log in or register to add a comment.