Question

Find all angle measures in the diagram

Answer 1

Explanation:

Angles 3x+5, 75 and x all together make up 180 degrees (review "straight angles").

Thus, 3x + 5 + 75 + x = 180

Simplifying the left side, we get:

4x + 80 = 180, so that 4x = 100, and x = 25.

<KJL = 3(25) + 5 = 80 degrees

<NJM = 25 degrees

<MJL = 75 degrees

Answer 2

The measures of the angles are:

`\[ \angle KJL = 100^\circ, \quad \angle NJM = 155^\circ, \quad \angle MJL = 105^\circ \]`

Given that KJN is a straight line, we know that the sum of the angles along this line is
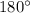`\( 180^\circ \)`. Therefore, we can express this relationship as:

`\[ \angle LJK + \angle LJM + \angle MJN = 180^\circ \]`

Now, substitute the given values:

(3x + 5) + 75 + x = 180

Combine like terms:

4x + 80 = 180

Subtract 80 from both sides:

4x = 100

Divide by 4:

x = 25

Now that we have the value of x, we can find each angle:

1. Angle KJL (by angle sum property in triangle KJL):

`\[ \angle KJL = 180^\circ - \angle LJK = 180^\circ - (3x + 5) = 180^\circ - (3 * 25 + 5) = 180^\circ - 80 = 100^\circ \]`

2. Angle NJM (by angle sum property in triangle NJM):

`\[ \angle NJM = 180^\circ - \angle MJN = 180^\circ - x = 180^\circ - 25 = 155^\circ \]`

3. Angle MJL (by angle sum property in triangle MJL):

`\[ \angle MJL = 180^\circ - \angle LJM = 180^\circ - 75 = 105^\circ \]`

Therefore, the measures of the angles are:

`\[ \angle KJL = 100^\circ, \quad \angle NJM = 155^\circ, \quad \angle MJL = 105^\circ \]`