1. Compare the series to the divergent p-series,

Use the limit comparison test: we have
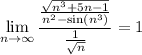
so the given series also diverges.
Breakdown for the limit:
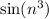 oscillates between -1 and 1, so its contribution to the denominator
oscillates between -1 and 1, so its contribution to the denominator
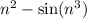 is neglible and we can replace it with
is neglible and we can replace it with
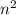 . In the square root in the numerator, the
. In the square root in the numerator, the
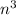 term dominates, so
term dominates, so
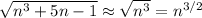 . So the given summand is approximated by
. So the given summand is approximated by

2. Let
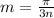 , so that
, so that
 as
as
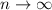 . Then
. Then
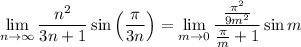
Recall that
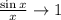 as
as
 . Rewrite the limit as
. Rewrite the limit as
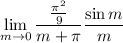
Then
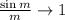 , and we're left with
, and we're left with
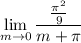
which is continuous at
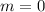 , giving a limit of
, giving a limit of
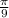 .
.