Answer:
v = 288.67 m/s
Step-by-step explanation:
Given that:
length of the separation I = 0.3
Resistance R = 50.0 ohms
Magnetic field B = 0.100 T
Power dissipated = 1.50 W
In a simple circuit, The Emf voltage of the power dissipated can be determined via the expression:
Power =
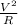
1.5 =
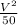
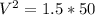
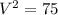
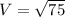
V = 8.660 V
From the Electromotive Force Emf ; we can calculate the speed of the bar
i.e Emf = B×v×l
8.660 = 0.1 × v × 0.3
v =
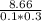
v = 288.67 m/s