Question

Media experts claim that daily print newspapers are declining because of Internet access. Listed​ below, from left to right and then top to​ bottom, are the numbers of daily print newspapers in a large region for a recent sequence of years. First find the​ median, then test for randomness of the numbers above and below the median using alphaequals0.05. What do the results​ suggest?

Answer 1

(a) The median is 1478

(b) Null hypothesis H₀ : μ₁ = μ₂

Alternative hypothesis Hₐ : μ₁ > μ₂

(c) The test statistic
`t_(\alpha/2)` is 6.678155

(d) The p value is 1.2×10⁻¹¹

(e) We reject our null hypothesis H₀. In terms of the test, there is sufficient evidence to suggest that there is a trend in the numbers of daily newspaper because the probability for a decrease in the numbers is very high

Explanation:

(a) Here we have the data as follows;

1623 1586 1574 1554 1544 1531 1519 1513 1491 1484 1478 1471 1458 1456 1458 1453 1438 1428 1405 1395 1377

The median is = 1478

Therefore we have above the median

1623 1586 1574 1554 1544 1531 1519 1513 1491 1484

The mean,
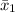`\bar{x}_(1)`= 1541.9

Standard deviation, σ₁ = 41.38224257

n₁ = 10

Below the median

1471 1458 1456 1458 1453 1438 1428 1405 1395 1377

The mean,
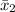`\bar{x}_(2)}`= 1433.9

Standard deviation, σ₂ = 30.04812806

n₂ = 10

(b) Null hypothesis H₀ : μ₁ = μ₂

Alternative hypothesis Hₐ : μ₁ > μ₂

(c) The formula for t test is given by;

`t_(\alpha/2) =\frac{(\bar{x}_(1)-\bar{x}_(2))}{\sqrt{(\sigma_(1)^(2) )/(n_(1))-(\sigma _(2)^(2))/(n_(2))}}`

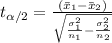
df = 10 - 1 = 9, α = 0.05

Therefore, the test statistic
`t_(\alpha/2)` = 6.678155

(d) The p value from statistical relations is Probability p = 1.2×10⁻¹¹

Critical z at 5% confidence level = 1.645

Since P << 0.05, e reject the

(e) Therefore, we reject our null hypothesis H₀. In terms of the test, there is sufficient evidence to suggest that there is a trend in the numbers of daily newspaper because the probability for a decrease in the numbers is very high.