Question

In a study, 42% of adults questioned reported that their health was excellent. A researcher wishes to study the health of people living close to a nuclear power plant. Among 11 adults randomly selected from this area, only 3 reported that their health was excellent. Find the probability that when 11 adults are randomly selected, 3 or fewer are in excellent health.

Answer 1

The probability that when 11 adults are randomly selected, 3 or fewer are in excellent health is 0.6483.

Explanation:

The random variable X can be defined as the number of adults questioned reported that their health was excellent.

A random sample of n = 11 adults are selected randomly selected from an area a nuclear power plant.

Of these 11 adults X = 3 reported that their health was excellent.

The proportion of adults who reported that their health was excellent is:

`p=(X)/(n)=(3)/(11)`

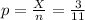
An adult's health condition is not affected by others, i.e. they are independent.

The random variable X follows a Binomial distribution with parameters n = 11 and p =
`(3)/(11)`.

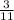The probability mass function of X is:

`P(X=x)={11\choose x}((3)/(11))^(x)(1-(3)/(11))^(11-x);\ x=0,1,2,3...`

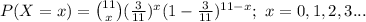
Compute the probability that when 11 adults are randomly selected, 3 or fewer are in excellent health as follows:

P (X ≤ 3) = P (X = 0) + P (X = 1) + P (X = 2) + P (X = 3)

`=\sum\limits^(3)_(x=0){{11\choose x}((3)/(11))^(x)(1-(3)/(11))^(11-x)}\\=0.02998+0.12385+0.23254+0.26197\\=0.64834\\\approx 0.6483`

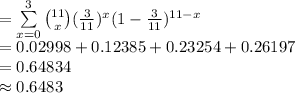
Thus, the probability that when 11 adults are randomly selected, 3 or fewer are in excellent health is 0.6483.