Question

The average gasoline price of one of the major oil companies in Europe has been $1.25 per liter. Recently, the company has undertaken several efficiency measures in order to reduce prices. Management is interested in determining whether their efficiency measures have actually reduced prices. A random sample of 49 of their gas stations is selected and the average price is determined to be $1.20 per liter. Furthermore, assume that the standard deviation of the population is $0.14.

a) Compute the standard error

b) Compute the test statistic

c) What is the p-value?

d) Develop appropriate hypothesis such as the reject of the null will support the contention that management efficiency measures had reduce gas prices in Europe.

e) At α = 0.05, what is your conclusion? Use critical value approach

f) Repeat the preceding hypothesis test using the p-value approach.

Answer 1

a)
`SE= (\sigma)/(√(n))= (0.14)/(√(49))= 0.02`

b)
`t =(1.20-1.25)/(0.02)= -2.5`

c)
`p_v =P(z<-2.5) =0.00621`

d) Null hypothesis:
`\mu \geq 1.25`

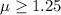
Alternative hypothesis:
`\mu<1.25`

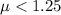
e) For this case we need to find a critical value who accumulate 0.05 of the area in the left at the normal standard distribution and we got:

`z_(crit)= -1.64`

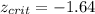
Since our calculated value is lower than the critical value we have enough evidence to reject the null hypothesis at the significance level provided of 5%

f)
`p_v = P(z<-2.5) = 0.00621`

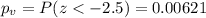
Since the p value is lower than the significance level we have enough evidence to reject the null hypothesis at 5% of significance. Same result with the critical value approach.

Explanation:

For this case we want to test is the true mean for the gasoline prices are significantly reduced from $1.25 per liter, so then the system of hypothesis are:

Null hypothesis:
`\mu \geq 1.25`

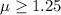
Alternative hypothesis:
`\mu<1.25`

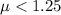
Part a

The standard error for this case is given by:

`SE= (\sigma)/(√(n))= (0.14)/(√(49))= 0.02`

Part b

The statistic for this hypothesis is given by:

`t = (\bar X -\mu)/(SE)`

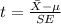
And replacing the info provided we got:

`t =(1.20-1.25)/(0.02)= -2.5`

Part c

Since we are conducting a left tailed test the p value would be given by:

`p_v =P(z<-2.5) =0.00621`

And we can use the following excel code to find it:

=NORM.DIST(-2.5,0,1,TRUE)

Part d

Null hypothesis:
`\mu \geq 1.25`

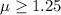
Alternative hypothesis:
`\mu<1.25`

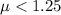
Part e

For this case we need to find a critical value who accumulate 0.05 of the area in the left at the normal standard distribution and we got:

`z_(crit)= -1.64`

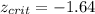
Since our calculated value is lower than the critical value we have enough evidence to reject the null hypothesis at the significance level provided of 5%

Part f

`p_v = P(z<-2.5) = 0.00621`

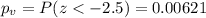
Since the p value is lower than the significance level we have enough evidence to reject the null hypothesis at 5% of significance. Same result with the critical value approach.