Answer:
The probability that the quality control manager will shut down the machine is 0.0002.
Explanation:
We are given that a machine is used to fill Apple Juice bottles with juice. The machine has a known standard deviation of σ = 0.05 liters. The target mean fill volume is µ = 2.0 liters.
A quality control manager obtains a random sample of 50 bottles. He will shut down the machine if the sample of these 50 bottles is less than 1.95 or greater than 2.1.
Let
 = sample mean fill volume
= sample mean fill volume
The z score probability distribution for sample mean is given by;
Z =
 ~ N(0,1)
~ N(0,1)
where,
 = population mean fill volume = 2 liters
= population mean fill volume = 2 liters
 = standard deviation = 0.05 liters
= standard deviation = 0.05 liters
n = sample of bottles = 50
Now, it is provided that he will shut down the machine if the sample of these 50 bottles is less than 1.95 or greater than 2.1.
- So, Probability that the sample of these 50 bottles is less than 1.95 is given by = P(
 < 1.95)
< 1.95)
P(
 < 1.95) = P(
< 1.95) = P(
 <
<
 ) = P(Z < -7.07) = 1 - P(Z
) = P(Z < -7.07) = 1 - P(Z
 7.07)
7.07)
= 1 - 0.9999 = 0.0001
- Probability that the sample of these 50 bottles is greater than 2.1 is given by = P(
 > 2.1)
> 2.1)
P(
 > 2.1) = P(
> 2.1) = P(
 >
>
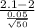 ) = P(Z > 14.14) = 1 - P(Z < 14.14)
) = P(Z > 14.14) = 1 - P(Z < 14.14)
= 1 - 0.9999 = 0.0001
Because the highest critical value in the z table is given as x = 4.40 for area of 0.99999.
Therefore, probability that the quality control manager will shut down the machine = 2
 0.0001 = 0.0002.
0.0001 = 0.0002.