Answer:
The pulling force applied to the cord connecting the larger flywheel is 30.88 N
Step-by-step explanation:
Given;
the radius of the smaller flywheel, r₁ = 21 cm
force on the cord of the smaller flywheel, F₁ = 50 N
the radius of the larger flywheel, r₂ = 34 cm
The torque on each flywheel is equal, since there is no rotation.
τ = Fr
where;
τ is torque on each flywheel
F is the force on the cord of each flywheel
r is the radius of each flywheel
F₁r₁ = F₂r₂
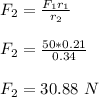
Therefore, the pulling force applied to the cord connecting the larger flywheel is 30.88 N