Question

A random sample of 9 fields of corn has a mean yield of 40.6 bushels per acre and standard deviation of 7.52 bushels per acre. Determine the 80% confidence interval for the true mean yield. Assume the population is approximately normal. Step 1. Find the critical value that should be used in constructing the confidence interval. Round your answer to three decimal places. Answer: ____________________ Step 2. Construct the 80% confidence interval. Round your answer to one decimal place. Answer: Lower endpoint: _______________ Upper endpoint: _______________

Answer 1

The critical value that should be used in constructing the confidence interval is -1.397 and 1.397.

80% confidence interval for the true mean yield is [37.1 bushels per acre, 44.1 bushels per acre].

Explanation:

We are given that a sample of 1584 third graders, the mean words per minute read was 35.7. Assume a population standard deviation of 3.3.

Firstly, the pivotal quantity for 80% confidence interval for the true mean is given by;

P.Q. =
`(\bar X-\mu)/((s)/(√(n) ) )` ~
`t_n_-_1`

where,
`\bar X` = sample mean yield = 40.6 bushels per acre

s = sample standard deviation = 7.52 bushels per acre

n = sample of fields of corn = 9

`\mu` = true mean yield

Here for constructing 80% confidence interval we have used One-sample t test statistics as we don't know about the population standard deviation.

So, 99% confidence interval for the true mean yield,
`\mu` is ;

P(-1.397 <
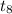`t_8` < 1.397) = 0.80 {As the critical value of t at 8 degree of

of freedom are -1.397 & 1.397 with P = 10%}

P(-1.397 <
`(\bar X-\mu)/((s)/(√(n) ) )` < 1.397) = 0.80

P(
`-1.397 * {(s)/(√(n) ) }` <
`{\bar X-\mu}` <
`1.397 * {(s)/(√(n) ) }` ) = 0.80

P(
`\bar X-1.397 * {(s)/(√(n) ) }` <
`\mu` <
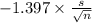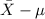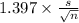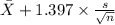`\bar X+1.397 * {(s)/(√(n) ) }` ) = 0.80

80% confidence interval for
`\mu` = [
`\bar X-1.397 * {(s)/(√(n) ) }` ,
`\bar X+1.397 * {(s)/(√(n) ) }` ]

= [
`40.6-1.397 * {(7.52)/(√(9) ) }` ,
`40.6+1.397 * {(7.52)/(√(9) ) }` ]

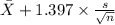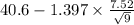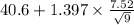= [37.1 , 44.1]

Therefore, 80% confidence interval for the true mean yield is [37.1 bushels per acre, 44.1 bushels per acre].