Answer:
The probability it will take a resident of the city between 17.24 and 42.13 minutes to travel to work is 0.3046.
Explanation:
The random variable X can be defined as the travel times to get to work.
The expected travel time is, μ = 38.3 minutes.
The distribution of random variable X can be defined as the distribution of time interval between which a person reaches their work place at a constant average rate.
This implies that X follows an Exponential distribution with parameter,
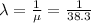 .
.
The probability density function of X is:
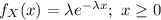
Compute the probability it will take a resident of the city between 17.24 and 42.13 minutes to travel to work as follows:
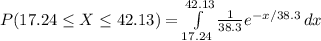
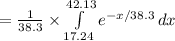

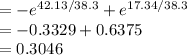
Thus, the probability it will take a resident of the city between 17.24 and 42.13 minutes to travel to work is 0.3046.