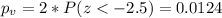Question

A study by Consumer Reports showed that 64% of supermarket shoppers believe supermarket brands to be as good as national name brands. To investigate whether this result applies to its own product, consider the hypothesis testing with H subscript 0 colon space p space equals space 0.64 space space space v s. space H subscript a colon space p space not equal to 0.64If a sample of 100 shoppers showed 52 stating that the supermarket brand was as good as the national brand, what is the p-value

Answer 1

`z=\frac{0.52 -0.64}{\sqrt{(0.64(1-0.64))/(100)}}=-2.5`

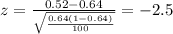
The p value for this case is given by:

`p_v =2*P(z<-2.5)=0.0124`

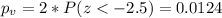
Explanation:

Data given and notatio

n=100 represent the random sample selected

X=52 represent the shoppers stating that the supermarket brand was as good as the national brand

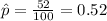`\hat p=(52)/(100)=0.52` estimated proportion of stating that the supermarket brand was as good as the national brand

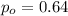`p_o=0.64` is the value that we want to test

z would represent the statistic

`p_v` represent the p value

System of hypothesis

We need to conduct a hypothesis in order to test the claim that the true proportion of shoppers stating that the supermarket brand was as good as the national brand is 0.64 or not, then the system of hypothesis are.:

Null hypothesis:
`p=0.64`

Alternative hypothesis:
`p \\eq 0.64`

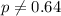
The statistic is given by:

`z=\frac{\hat p -p_o}{\sqrt{(p_o (1-p_o))/(n)}}` (1)

Calculate the statistic

The statistic is given by:

`z=\frac{0.52 -0.64}{\sqrt{(0.64(1-0.64))/(100)}}=-2.5`

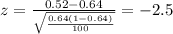
Statistical decision

The p value for this case is given by:

`p_v =2*P(z<-2.5)=0.0124`