Answer:
The probability of wining the jackpot = 1.180×10⁻⁸
Explanation:
The number of possible ways to pick first 5 different integers between 1 and 50 is given by
n₁ = ⁵⁰C₅
n₁ = 2118760
The number of possible ways to pick 6th integer between 1 and 40 is given by
n₂ = ⁴⁰C₁
n₂ = 40
The number of ways to correctly guess the first five integers is given by
p₁ = ⁵C₅
p₁ = 1
The number of ways to correctly guess the 6th integer is given by
p₂ = ¹C₁
p₂ = 1
The probability of wining the jackpot where the first five numbers picked match the five numbers drawn and the sixth number picked matches the sixth number drawn is given by
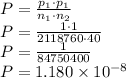
Therefore, the probability of wining the jackpot is 1.180×10⁻⁸