Question

Use a one-sample t ‑test, based on the data below, to test the null hypothesis H0:µ=100.63 against the alternative hypothesis H1:µ>100.63 . The sample has a mean of x⎯⎯⎯=101.09 and a standard deviation of s=0.4887 . 100.68,101.23,100.82,101.15,100.96,100.70,102.09 Calculate the standard error (SE) and the t ‑statistic for this test. Give the standard error to four decimal places and t to three decimal places.

Answer 1

The standard error (SE) is 0.1847.

The t-statistic for this test is 2.490.

Explanation:

We are given that the sample has a mean of
`\bar X` = 101.09 and a standard deviation of s = 0.4887 .

Also, the 7 sample values are also given.

Let
`\mu` = population mean.

So, Null Hypothesis,
`H_0` :
`\mu` = 100.63

Alternate Hypothesis,
`H_1` :
`\mu` > 100.63

The test statistics that would be used here One-sample t test statistics as we don't know about population standard deviation;

T.S. =
`(\bar X-\mu)/((s)/(√(n) ) )` ~
`t_n_-_1`

where,
`\bar X` = sample mean = 101.09

s = sample standard deviation = 0.4887

n = sample values = 7

The Standard Error (SE) is given by =
`(s)/(√(n) )` =
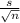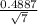`(0.4887)/(√(7) )` = 0.1847

So, test statistics =
`(101.09-100.63)/((0.4887)/(√(7) ) )` ~
`t_6`

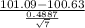= 2.490

The value of t test statistics is 2.490.