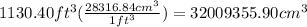Question

What is the volume of the oblique cylinder rounded to the nearest cm^3?

Answer 1

Answer:
`32009355.90cm^3`

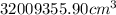
Explanation:

Formula:
`V=Bh\\`

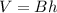
Since the base is a circumference, we can replace B for the area of a circumference formula.

`V=\pi r^2h`

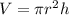
What we have here is 12 ft as the diameter of the circumference. A diameter is twice the radius, therefore we can conclude that if we take the diameter and divide it by 2, it will give us the radius.

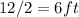`12/2=6ft` this is the radius.

Now plug all this information into your formula.

`V=(3.14)(6ft)^2(10ft)\\V=(3.14)(36ft^2)(10ft)\\V=1130.40ft^3`

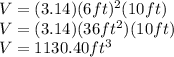
Our values are in feet but the question requires cm. Let's convert from
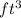`ft^3` to
`cm^3`.

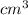Normally, our conversion factors are raised to the power of 1, but in this case it's raised to the power of 3. So, first let's see how many cm are 1 ft.

`1ft=30.48cm`

Here is where magic comes. We can raise both to the power of 3, in order to find the cubic ft and cm that we need as our conversion factors.

`(1ft)^3=(30.48cm)^3\\1ft^3=28316.8cm^3`

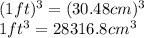
Now we have our conversion factors.

`1130.40ft^3((28316.84cm^3)/(1ft^3))=32009355.90cm^3`