Explanation:
Hello there, I think your question missed key information, allow me to add in and hope it will fit the orginal one.
Let assume the side of the cube is a units
=>The surface area of the cude is:
- A =

Given that The bottom of the cake and the area where the two cakes meet is not frosted.
=> the are of a cube that is frosted in the first layer
A' = A -
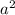
=
 -
-
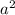
=5
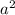
=> the are of a cube that is frosted in the second layer
A'' =
 -
-
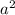 -
-
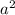 (the bottom and the top of it are not frosted)
(the bottom and the top of it are not frosted)
= 4
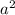
=> the total the area of the cake that is frosted is:
TA = A' + A''
= 5
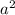 + 4
+ 4
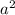
= 9
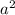 square units
square units
To solve this type of question, you just need to substitute the actual side of the cube into the expression 9
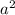 . Hope it will find you well.
. Hope it will find you well.