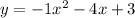Answer:
B. -1
Explanation:
If we have a parabola whose equation is:
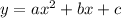
The line of symmetry is calculated as:

Now, we have the equation
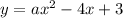 and the line of symmetry is
and the line of symmetry is

Where:
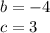
So, we can replace
 by -4 and
by -4 and
 by -2 and solve for
by -2 and solve for
 using the following equation as:
using the following equation as:
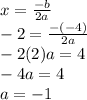
It means that the equation of the parabola is equal to: