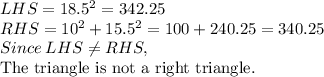Question

given that a triangle LMN has side inches of 18.5 inches, 10, inches, and 15.5 inches, prove triangle LMN is aright triangle.

Answer 1

LMN is not a right triangle.

Explanation:

To verify that the lengths of a triangle for a right triangle, all we need to do is to check it they satisfy the Pythagoras Theorem.

Pythagoras Theorem:
`Hypotenuse^2=Opposite^2+Adjacent^2`

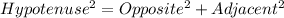
The Hypotenuse is always the longest side.

Since no angle is given, the two other sides can be alternated.

Given lengths of triangle LMN: 18.5 inches, 10, inches, and 15.5 inches

`LHS=18.5^2=342.25\\RHS=10^2+15.5^2=100+240.25=340.25\\Since \: LHS\\eq RHS,\\$The triangle is not a right triangle.`