Question

According to a Pew Research Center, in May 2011, 35% of all American adults had a smart phone (one which the user can use to read email and surf the Internet). A communications professor at a university believes this percentage is higher among community college students.

She selects 300 community college students at random and finds that 120 of them have a smart phone. In testing the hypotheses: H0: p = 0.35 versus Ha: p > 0.35, she calculates the test statistic as Z = 1.82.

Use the Normal Table to help answer the p-value part of this question.

Click here to access the normal table.

1. There is enough evidence to show that more than 35% of community college students own a smart phone (P-value = 0.034).

2. There is enough evidence to show that more than 35% of community college students own a smart phone (P-value = 0.068).

3. There is not enough evidence to show that more than 35% of community college students own a smart phone (P-value = 0.966).

4. There is not enough evidence to show that more than 35% of community college students own a smart phone (P-value = 0.034).

Answer 1

`p_v =P(z>1.82)=0.034`

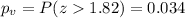
Assuming a standard significance level of
`\alpha=0.05` the best conclusion for this case would be:

4. There is not enough evidence to show that more than 35% of community college students own a smart phone (P-value = 0.034).

Because
`p_v <\alpha`

If we select a significance level lower than 0.034 then the conclusion would change.

Explanation:

Data given

n=300 represent the random sample taken

X=120 represent the people who have a smart phone

`\hat p=(120)/(300)=0.4` estimated proportion of people who have a smart phone

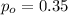`p_o=0.35` is the value that we want to test

z would represent the statistic (variable of interest)

`p_v` represent the p value (variable of interest)

System of hypothesis

We need to conduct a hypothesis in order to test the claim that the true proportion of people who have a smart phone is higher than 0.35, the system of hypothesis are.:

Null hypothesis:
`p\leq 0.35`

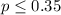
Alternative hypothesis:
`p > 0.35`

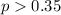
The statistic is given by:

`z=\frac{\hat p -p_o}{\sqrt{(p_o (1-p_o))/(n)}}` (1)

Calculate the statistic

Since we have all the info requires we can replace in formula (1) like this:

`z=\frac{0.4 -0.35}{\sqrt{(0.35(1-0.35))/(300)}}=1.82`

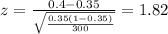
Statistical decision

Since is a right tailed test the p value would be:

`p_v =P(z>1.82)=0.034`

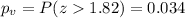
Assuming a standard significance level of
`\alpha=0.05` the best conclusion for this case would be:

4. There is not enough evidence to show that more than 35% of community college students own a smart phone (P-value = 0.034).

Because
`p_v <\alpha`

If we select a significance level lower than 0.034 then the conclusion would change.