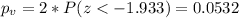Question

A publisher reports that 45% of their readers own a laptop. A marketing executive wants to test the claim that the percentage is actually different from the reported percentage. A random sample of 370 found that 40% of the readers owned a laptop. determine the p-value of the test statistic

Answer 1

`z=\frac{0.40 -0.45}{\sqrt{(0.45(1-0.45))/(370)}}=-1.933`

`p_v =2*P(z<-1.933)=0.0532`

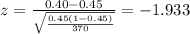
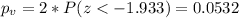
Explanation:

Information given

n=370 represent the sample selected

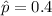`\hat p=0.4` estimated proportion of readers owned a laptop

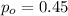`p_o=0.45` is the value that we want to test

z would represent the statistic

`p_v` represent the p value

Creating the hypothesis

We need to conduct a hypothesis in order to test if the true proportion of readers owned a laptop is different from 0.45, the system of hypothesis are:

Null hypothesis:
`p=0.45`

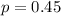
Alternative hypothesis:
`p \\eq 0.45`

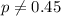
The statistic is:

`z=\frac{\hat p -p_o}{\sqrt{(p_o (1-p_o))/(n)}}` (1)

Replacing we got:

`z=\frac{0.40 -0.45}{\sqrt{(0.45(1-0.45))/(370)}}=-1.933`

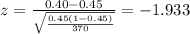
Calculating the p value

We have a bilateral test so then the p value would be:

`p_v =2*P(z<-1.933)=0.0532`