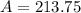Answe
Given,
f(x) = 49 − x² from x = 1 to x = 7
n = 4
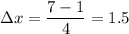
For x= 1
f(x₀) = 49 - 1^2 = 48
x = 2.5
f(x₁) = 42.75
x = 4
f(x₂) = 49 - 4^2 = 33
x = 5.5
f(x₃) = 49 - 5.5^2 = 18.75
x = 7
f(x₄) = 49 - 7^2 = 0
We have to evaluate the function on therigh hand point
![A = \Delta x [f(x_1)+f(x_2)+f(x_3)+f(x_4)]](https://img.qammunity.org/2021/formulas/mathematics/middle-school/qf8i3awnjjcpwt4kyd5i74mo8fh91akw5l.png)
![A = 1.5 [42.75+33+18.75+0]](https://img.qammunity.org/2021/formulas/mathematics/middle-school/lndqoim8349g4m1igqckzuictbbdgfngb2.png)
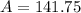
For Area for left hand sum
![A = \Delta x [f(x_0)+f(x_1)+f(x_2)+f(x_3)]](https://img.qammunity.org/2021/formulas/mathematics/middle-school/3d58g9b6xv8xvrbens12mkcabg5zcrphya.png)
![A = 1.5 [48+42.75+33+18.75]](https://img.qammunity.org/2021/formulas/mathematics/middle-school/zkxlw51329lm21zcuhezshs0cvd0z5ipct.png)