Question

Find the 90% confidence interval for the population standard deviation given the following.

n = 51, x = 11.49, s = 2.34 and the distribution is normal.

a. 1.92 < σ < 3.25
b. 2.01 < σ < 2.81
c. 1.37 < σ < 1.91
d. 10.28 < σ < 14.32

Answer 1

b. 2.01 < σ < 2.81

Explanation:

The 90% confidence interval for the population standard deviation is calculated as:

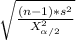`\sqrt{((n-1)*s^(2) )/(X^2_(\alpha/2 ) ) }` < σ <
`\sqrt{((n-1)*s^(2) )/(X^2_(1-\alpha/2 ) ) }`

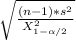
Where n is equal to 51, s is equal to 2.34,
`\alpha` is 10%,
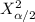`X^(2) _(\alpha /2)` is the value for the Chi squared distribution with n-1 degrees of freedom that has a probability of
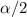`\alpha /2` in the right tail and
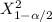`X^(2) _(1-\alpha /2)` is the value for the Chi squared distribution with n-1 degrees of freedom that has a probability of
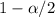`1-\alpha /2` in the right tail.

So, replacing n by 51, s by 2.34,
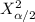`X^(2) _(\alpha /2)` by 67.50 and
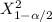`X^(2) _(1-\alpha /2)` by 34.76, we get that the The 90% confidence interval is equal to:

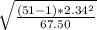`\sqrt{((51-1)*2.34^(2) )/(67.50) } }` < σ <
`\sqrt{((51-1)*2.34^(2) )/(34.76) } }`

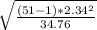
2.01 < σ < 2.81