Question

Find the equation of a line that passes through the points (-4,-2) and (6, 3).

Answer 1

`y = (x)/(4)`

Explanation:

we find the slope which also represented as m by the formula

`m = ((y2 - y1))/((x2 - x1))`

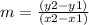
where from point (-4, -2) the x1 is -4 and the y1 is -2 and also from point (6, 3) the x2 is 6 and the y2 is 3.

so we substitute

`((3 - ( - 2)))/((6 - ( - 4))) = ((3 + 2))/((6 + 4)) = (5)/(10) = (1)/(2)`

`m = (1)/(2)`

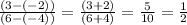
after we have the slope, we calculate the equation of the line by using the formula

`(y - y1) = m(x - x1)`

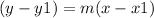
but for now, we only have y1, m and x1 so can substitute

`(y - ( - 2)) = (1)/(2) (x - ( - 4))`

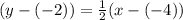
we have to get rid of the fraction 1/2 so we multiply it through by the denominator 2

`2(y + 2) = 2 * (1)/(2)(x + 4)`

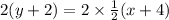
we get

`2(y + 2) = 1 * (x + 4)`

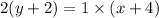
so now we expand the brackets

`2y + 4 = x + 4`

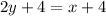
we make y stand alone by grouping liked terms.

`2y = x + 4 - 4`

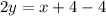
we get

`y = (x)/(4)`