1 Answer

Skypanther · Answer 1 · 2021-11-11T15:38:25+0000

Answer:

The length of the sides of the square is 9.0015

Explanation:

Given

The diagonal of a square = 12.73

Required

The length of its side

Let the length and the diagonal of the square be represented by L and D, respectively.

So that

D = 12.73

The relationship between the diagonal and the length of a square is given by the Pythagoras theorem as follows:

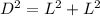
D^(2) = L^(2) + L^(2)

Solving further, we have

D^(2) = 2L^(2)

Divide both sides by 2

(D^(2))/(2) = (2L^(2))/(2)

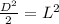
(D^(2))/(2) = L^2

Take Square root of both sides

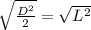
$\sqrt{(D^(2))/(2)} = √(L^2)$

$\sqrt{(D^(2))/(2)} = L$

Reorder

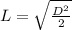
$L = \sqrt{(D^(2))/(2)}$

Now, the value of L can be calculated by substituting 12.73 for D

$L = \sqrt{(12.73^(2))/(2)}$

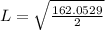
$L = \sqrt{(162.0529)/(2)}$

$L = \sqrt{{81.02645}$

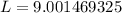
L = 9.001469325

L = 9.0015 (Approximated)

Hence, the length of the sides of the square is approximately 9.0015

Please log in or register to add a comment.