Question

A regular pentagon has an apothem of 7.3 inches and a perimeter of 53 inches. What is the area of the pentagon? Round your answer to the nearest whole number

Answer 1

Answer: The area of the pentagon is 194 square inches

Step-by-step explanation: The question has given us a regular pentagon which means all five sides are equal and measure the same length. The perimeter has also been given as 53 inches. The perimeter of a plane shape is simply the measurement around all its sides. So if the perimeter of a regular pentagon is given as 53, then each side would measure, 53 divided by 5 and that would result in 10.6 inches per side. Also the apothem, which is the line that is drawn from the center and touches each side at a perpendicular measures 7.3 inches. To calculate the area of the pentagon we shall apply the formula given as follows;

Area = 1/2 x a x p

Where a is the apothem, and p (perimeter) is derived as number of sides times length of a side. P therefore is calculated as 5 times 10.6, which results in 53.

Area = 1/2x 7.3 x 53

Area = 1/2 x 386.9

Area = 193.45

Area ≈ 194 (to the nearest whole number)

The area of the pentagon therefore is 194 square inches (rounded to the nearest whole number)

Answer 2

Area pf the regular pentagon is 193
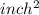`inch^(2)` to the nearest whole number

Explanation:

In this question, we are tasked with calculating the area of a regular pentagon, given the apothem and the perimeter

Mathematically, the area of a regular pentagon given the apothem and the perimeter can be calculated using the formula below;

Area of regular pentagon = 1/2 × apothem × perimeter

From the question, we can identify that the value of the apothem is 7.3 inches, while the value of the perimeter is 53 inches

We plug these values into the equation above to get;

Area = 1/2 × 7.3× 53 = 386.9/2 = 193.45 which is 193
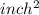`inch^(2)` to the nearest whole number