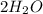Question

Stoichiometry!

Please note:
- Use 6.022x1023 for avogadro’s number
- Ignore sig figs and do not round the final answer.
- Keep it to 1 decimal place.

Answer 1

a) 13.2 moles
`2H_(2)O`

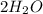
b) 79.33 grams of
`2H_(2)O`

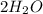
Step-by-step explanation:

First, we'll need to balance the equation

`H_(2(g)) + O_(2(g))` →
`H_(2)O_((g))`

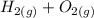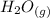
There are 2 (O) on the left and only one on the right, so we'll add a 2 coefficient to the right.

`H_(2(g)) + O_(2(g))` →
`2H_(2)O_((g))`

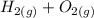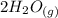
Now there are 4 (H) on the right and only 2 on the left, so we'll add a 2 coefficient to the (
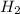`H_(2)`) on the left.

`2H_(2(g)) + O_(2(g))` →
`2H_(2)O_((g))`

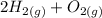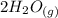
The equation is now balanced.

a) This can be solved with a simple mole ratio.

4.6 moles
`O_(2)` ×
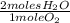`(2 moles H_(2)O)/(1 mole O_(2))` = 13.2 moles
`2H_(2)O`

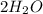
b) This problem is solved the same way!

2.2 moles
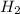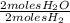`H_(2)` ×
`(2 moles H_(2)O)/(2 moles H_(2))` = 2.2 moles
`2H_(2)O`

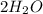
However, this problem wants the mass of
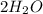`2H_(2)O`, not the moles.

The molecular weight of
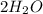`2H_(2)O` is the weight of 4 (H) molecules and 2 (O) molecules (found on the periodic table). So,

4(1.008) + 2(15.999) = 36.03 g/mol

2.2 moles
`2H_(2)O` ×
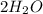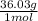`(36.03 g)/(1 mol)` = 79.33 grams of
`2H_(2)O`