Correction To the Question
- The dimensions of the 6 blocks stacked are
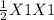
- The dimensions of the 9 blocks stacked are
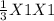 .
.
Answer:
- (A)Six of the one-half cubes would have a volume of 3 cubic units.
- (D)Three of the one-third cubes will make 1 unit cube.
- (E)Two of the one-half cubes will make 1 unit cube.
- (F)Both stacks will have a volume of 3 cubic units.
Step-by-step explanation:
Marissa claims that stacking 6 blocks with dimensions of
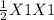 will give the same volume as stacking 9 blocks with dimensions of
will give the same volume as stacking 9 blocks with dimensions of
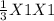 .
.
First, we examine the volume of each block.
Block 1
The dimensions are:
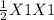
Volume of 1 block =
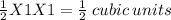
Volume of 6 blocks of dimension
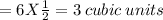
Block 2
The dimensions are:
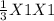
Volume of 1 block =
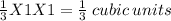
Volume of 9 blocks of dimension

The following statements out of her claim are true:
- (A)Six of the one-half cubes would have a volume of 3 cubic units.
- (D)Three of the one-third cubes will make 1 unit cube.
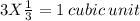
- (E)Two of the one-half cubes will make 1 unit cube.
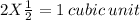
- (F)Both stacks will have a volume of 3 cubic units.